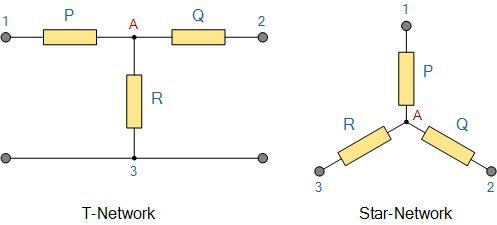
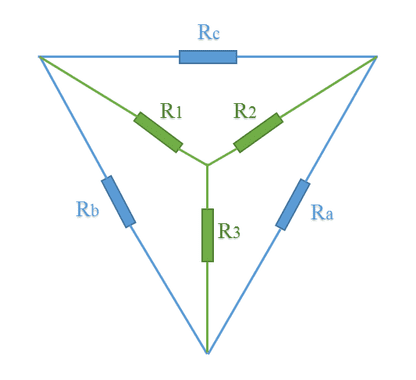
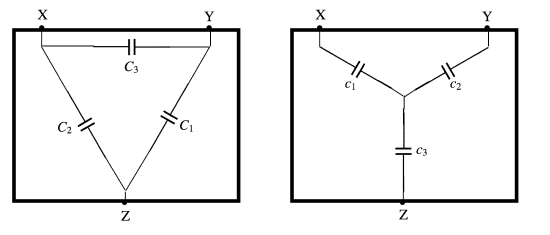
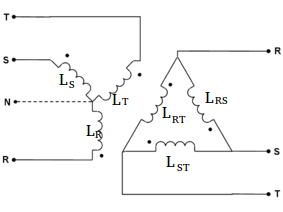
In addition to parallel and series, impedances also can make star and delta connections. Also are shown the transformations.
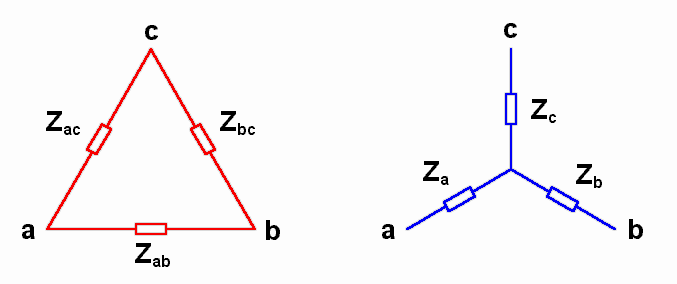
Star and delta connections
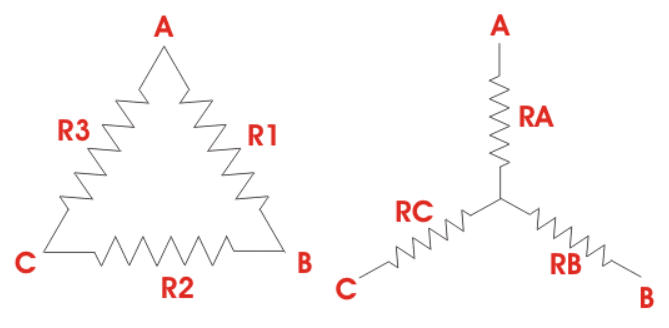 On the left, resistors linked in delta and on the right, star. Source: electrical4u.
On the left, resistors linked in delta and on the right, star. Source: electrical4u.Some sources label delta connection as pi and star as T.
Star-delta transformation (Y-Δ)
Equations to transform a star configuration to delta.
Ra=R1R1R2+R1R3+R2R3
Rb=R2R1R2+R1R3+R2R3
Rc=R3R1R2+R1R3+R2R3
Delta-star transformation (Δ-Y)
Equations for (Δ-Y) transformation.
R1=Ra+Rb+RcRbRc
R2=Ra+Rb+RcRaRc
R3=Ra+Rb+RcRaRb
If all resistors are equal, the equations become much simpler. Considering RY as resistor value in star and RΔ of resistor in delta.
RY=3RΔ
RΔ=3⋅RY
What if instead of resistors, we have capacitors and coil?
Demonstrating the equation of (Δ-Y) transformation for capacitors.
c11=C11+C21+C31C2C31
c1=C1C2C3+C3C1+C1C2
c2=C2C2C3+C3C1+C1C2
c3=C3C2C3+C3C1+C1C2
Equations for inverse transformation (Y-Δ).
C11=c11c1c21+c1c31+c2c31
C1=c1+c2+c3c2c3
C2=c1+c2+c3c1c3
C3=c1+c2+c3c1c2
And for inductors, the calculations are similar to resistors.
Equivalent values from Y to Δ.
LRS=LTLRLS+LSLT+LRLT
LRT=LSLRLS+LSLT+LRLT
LST=LRLRLS+LSLT+LRLT
Equivalent values from Δ to Y.
LR=LRS+LRT+LSTLRSLRT
LS=LRS+LRT+LSTLRSLST
LT=LRS+LRT+LSTLSTLRT
And for impedances.
Conversion from Y to Δ.
Zac=ZbZaZc+ZaZb+ZbZc
Zab=ZcZaZc+ZaZb+ZbZc
Zbc=ZaZaZc+ZaZb+ZbZc
Conversion from Δ to Y.
Za=Zab+Zac+ZbcZabZac
Zb=Zab+Zac+ZbcZabZbc
Zc=Zab+Zac+ZbcZacZbc
3 problem examples
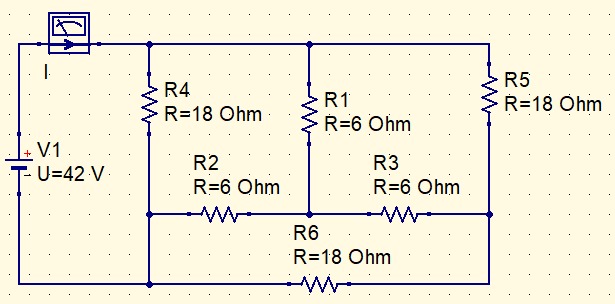
Let’s find the value of I current in this circuit.
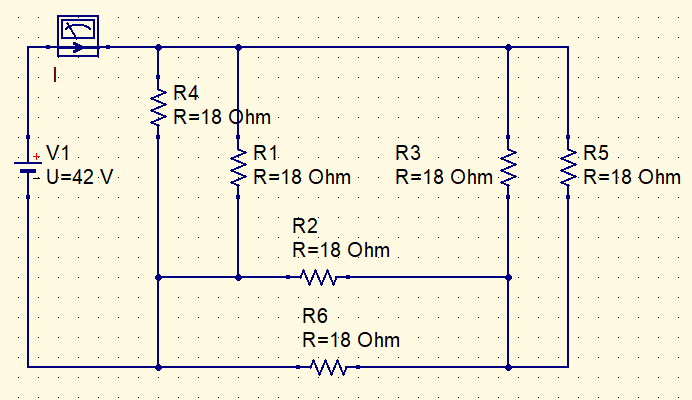
Converting internal star in delta.
RΔ=3⋅RY
RΔ=3⋅6=18Ω
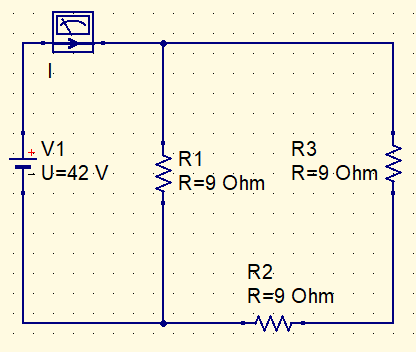
3 resistor pairs are in parallel. Just simplify the circuit to find I current.
Rt=9+189⋅18=6Ω
I=642=8A
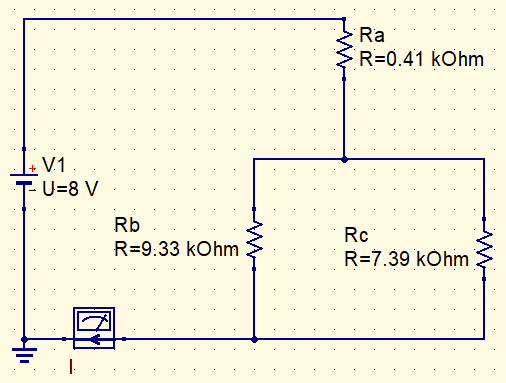
How to calculate I current in this circuit?
Replacing R1, R2 and R3 with (Δ-Y) tranformation.
Ra=4,7k+1,1k+6,8k4,7k⋅1,1k=12,65,17k=0,41kΩ
Rb=4,7k+1,1k+6,8k4,7k⋅6,8k=12,631,96k=2,53kΩ
Rc=4,7k+1,1k+6,8k1,1k⋅6,8k=12,67,48k=0,59kΩ
With simplified circuit, becomes easier to calculate current.
Rt=16,72k9,33k⋅7,39k=4,12kΩ
I=0,41k+4,12k8=1,76mA
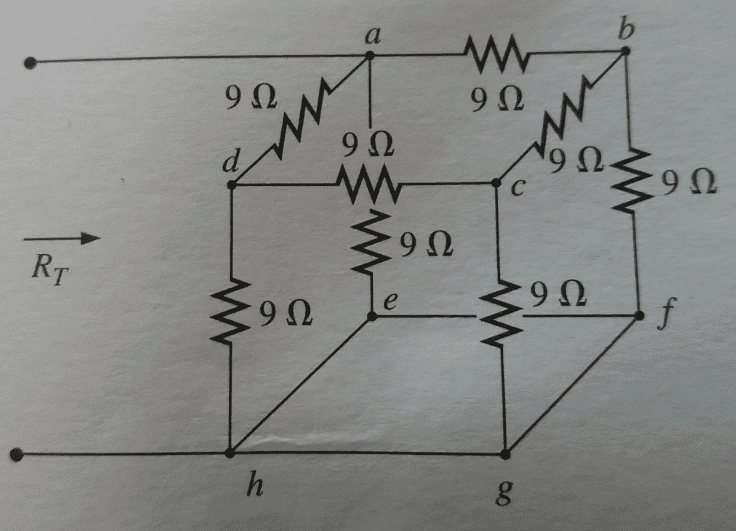
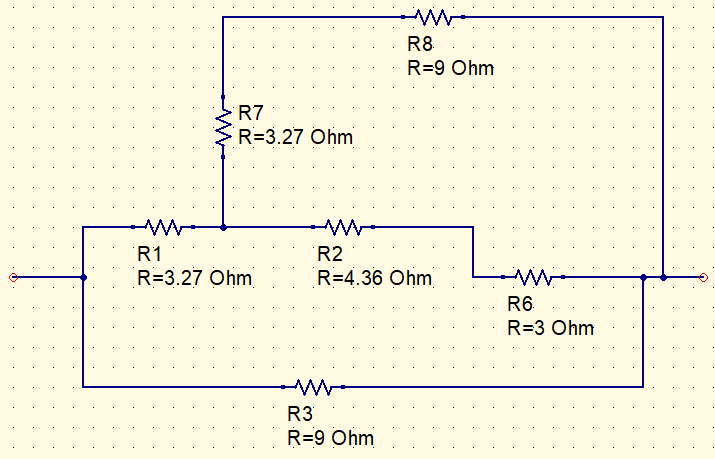
How to find total resistance of this resistor association?
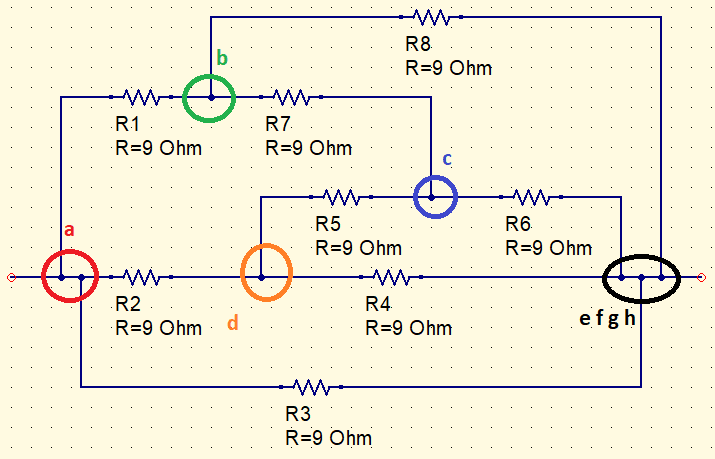
Putting this cube in a shape that allows to visualize a group of resistors for conversion.
RY=3RΔ=39=3Ω
Converting delta on the left in star.
Ra=Rb=R1+R2+R7R1⋅R2=339⋅12=3,27Ω
Rc=9+12+1212⋅12=4,36Ω
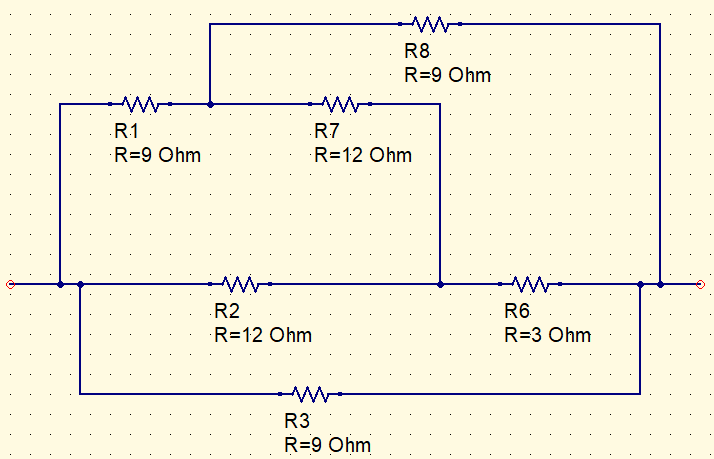
R7+R8=12,27Ω
R2+R6=7,36Ω
12,27+7,3612,27⋅7,36=4,6Ω
3,27+4,6=7,87Ω
Finally, the total resistance is:
Rt=9+7,879⋅7,87=4,19Ω